Introduction

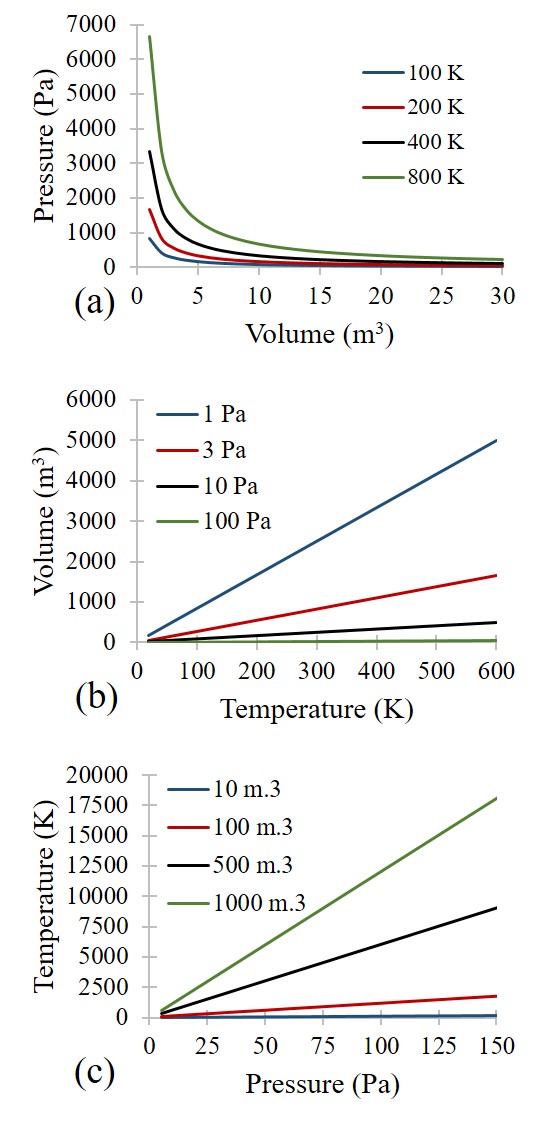
The Ideal Gas Law is a simple equation demonstrating the relationship between temperature, pressure, and volume for gases. These specific relationships stem from Charles’s Law, Boyle’s Law, and Gay-Lussac’s Law. Charles’s Law identifies the direct proportionality between volume and temperature at constant pressure, Boyle’s Law identifies the inverse proportionality of pressure and volume at a constant temperature, and Gay-Lussac’s Law identifies the direct proportionality of pressure and temperature at constant volume. Combined, these form the Ideal Gas Law equation: PV = NRT. P is the pressure, V is the volume, N is the number of moles of gas, R is the universal gas constant, and T is the absolute temperature.

The universal gas constant R is a number that satisfies the proportionalities of the pressure-volume-temperature relationship. R has different values and units that depend on the user’s pressure, volume, moles, and temperature specifications. Various values for R are on online databases, or the user can use dimensional analysis to convert the observed units of pressure, volume, moles, and temperature to match a known R-value. As long as the units are consistent, either approach is acceptable. The temperature value in the Ideal Gas Law must be in absolute units (Rankine [degrees R] or Kelvin [K]) to prevent the right-hand side from being zero, which violates the pressure-volume-temperature relationship. The conversion to absolute temperature units is a simple addition to either the Fahrenheit (F) or the Celsius (C) temperature: Degrees R = F + 459.67 and K = C + 273.15. 

For a gas to be “ideal” there are four governing assumptions:

1. The gas particles have negligible volume.
2. The gas particles are equally sized and do not have intermolecular forces (attraction or repulsion) with other gas particles.
3. The gas particles move randomly in agreement with Newton’s Laws of Motion.
4. The gas particles have perfect elastic collisions with no energy loss.

In reality, there are no ideal gases. Any gas particle possesses a volume within the system (a minute amount, but present nonetheless), which violates the first assumption. Additionally, gas particles can be of different sizes; for example, hydrogen gas is significantly smaller than xenon gas. Gases in a system do have intermolecular forces with neighboring gas particles, especially at low temperatures where the particles are not moving quickly and interact with each other. Even though gas particles can move randomly, they do not have perfect elastic collisions due to the conservation of energy and momentum within the system.[1][2][3]

While ideal gases are strictly a theoretical conception, real gases can behave ideally under certain conditions. Systems with either very low pressures or high temperatures enable real gases to be estimated as “ideal.” The low pressure of a system allows the gas particles to experience less intermolecular forces with other gas particles. Similarly, high-temperature systems allow for the gas particles to move quickly within the system and exhibit less intermolecular forces with each other. Therefore, for calculation purposes, real gases can be considered “ideal” in either low pressure or high-temperature systems.

The Ideal Gas Law also holds true for a system containing multiple ideal gases; this is known as an ideal gas mixture. With multiple ideal gases in a system, these particles are still assumed not to have any intermolecular interactions with one another. An ideal gas mixture partitions the total pressure of the system into the partial pressure contributions of each of the different gas particles. This allows for the previous ideal gas equation to be re-written:  Pi·V = ni·R·T. In this equation, Pi is the partial pressure of species i and ni are the moles of species i. At low pressure or high-temperature conditions, gas mixtures can be considered ideal gas mixtures for ease of calculation.

When systems are not at low pressures or high temperatures, the gas particles are able to interact with one another; these interactions greatly inhibit the Ideal Gas Law’s accuracy. There are, however, other models, such as the Van der Waals Equation of State, that account for the volume of the gas particles and the intermolecular interactions. The discussion beyond the Ideal Gas Law is outside the scope of this article.

Function

Despite other more rigorous models to represent gases, the Ideal Gas Law is versatile in representing other phases and mixtures. Christensen et al. performed a study to create calibration mixtures of oxygen, isoflurane, enflurane, and halothane. These gases are commonly used in anesthetics, which require accurate measurements to ensure the patient's safety. In this study, Christensen et al. compared the use of ideal gas assumption to more rigorous models to identify the partial pressures of each of the gases. The ideal gas assumptions had a 0.03% error for the calibration experiment. This study concluded that the error from the ideal gas assumption could be used to tune the calibration of the anesthetics, but the deviation itself was not appreciable to prevent use on patients.[4][5][6]

In addition to gaseous mixtures, the ideal gas law can model the behavior of certain plasmas. In a study by Oxtoby et al., the researchers found that dusty plasma particles could be modeled by ideal gas behaviors. The study suggests the reason for this similarity stems from low compression ratios of dusty plasma afforded the ideal gas behavior. While more complex models will need to be created, the plasma phases were accurately models were accurately represented by the Ideal Gas Law.

Ideal gases also have contributed to the study of surface tension in water. Sega et al. proved the ideal gas contribution to surface tension in water was not trivial but a rather finite amount. Sega et al. created a new expression that better represented the ideal gas contribution to the surface tension. This can allow for a more accurate representation of gas-liquid interfaces in the future.

The Ideal Gas Law and its behavior primarily serve as an initial step to obtaining information about a system. More complex models are available to describe a system accurately; however, should accuracy not be the main consideration, the Ideal Gas Law affords ease of calculation while providing physical insights into the system.[7][8]

Issues of Concern

The main issue of concern with the Ideal Gas Law is that it is not always accurate because there are no true ideal gases. The governing assumptions of the Ideal Gas Law are theoretical and omit many aspects of real gases. For example, the Ideal Gas Law does not account for chemical reactions that occur in the gaseous phase that could change the pressure, volume, or temperature of the system. This is a significant concern because the pressure can rapidly increase in gaseous reactions and quickly become a safety hazard. Other relationships, such as the Van der Waals Equation of State, are more accurate at modeling real gas systems.

Clinical Significance

The Ideal Gas Law presents a simple calculation to determine the physical properties of a given system and serves as a baseline calculation. As studied in Christensen et al., the Ideal Gas Law can be used to calibrate anesthetic mixtures with a nominal error. At high-altitude environments, the Ideal Gas Law would be more accurate for monitoring the pressure of gas flow into patients than at sea level. If there are significant temperature fluctuations, the pressure needed to deliver oxygen to a patient must be adjusted; the Ideal Gas Law can be used as an approximation. While more sophisticated calculations offer greater accuracy overall, the Ideal Gas Law can develop physician intuition when operating with real gases. 

Enhancing Healthcare Team Outcomes

All members of the interprofessional healthcare team, whether they be clinicians, nurses, anesthesia specialists, or anesthesia nurses, all need to have familiarity with the Ideal Gas Law and its application in medical care. Being facile with the formula and its application can prevent medical errors and optimize patient care in situations (e.g., anesthesia) where it is applicable. [Level 5]